Logarithmic Functions
A Logarithmic Function is an inverse function of an exponential function.
and
Logarithmic functions are widely used to represent quantities that spread out over a wide range (earthquake magnitude, sound level, etc).
Taking the logarithm of a number produces the exponent to which the base of the logarithm was originally raised.
Let’s go over how to graph logarithmic functions and how to solve logarithmic equations.
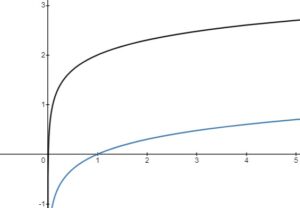
Graphing Logarithmic Functions
(base 10, from
)
|
|
|
| -1 | undefined |
| 0 | undefined |
| 1 | 0 |
| 2 | 0.3 |
| 3 | 0.477 |
There are no -intercept, as there is a Vertical Asymptote (VA) at
The -intercept is at (1,0)
Domain: { }
Range: { }
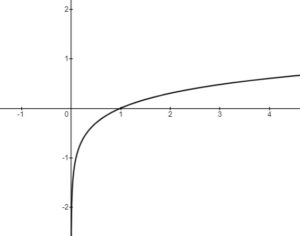
(base
, natural logarithm from
)
|
|
|
| -1 | undefined |
| 0 | undefined |
| 1 | 0 |
| 2 | 0.693 |
| 3 | 1.0986 |
There are no -intercept, as there is a Vertical Asymptote (VA) at
The -intercept is at (1,0)
Domain: { }

Transformations of Log Functions
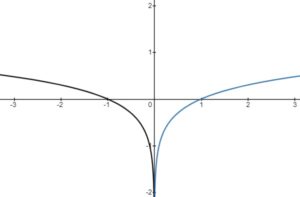
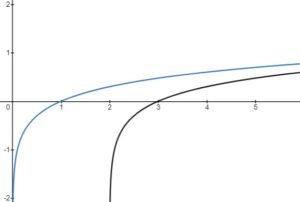
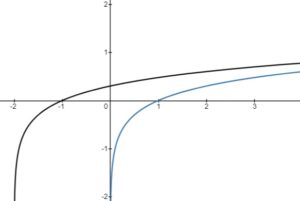
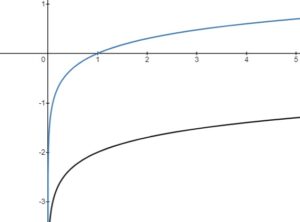
– vertical stretch or compression, reflection in the
-axis
– horizontal stretch or compression, reflection in the
-axis
– horizontal translation left or right
– vertical translation up or down
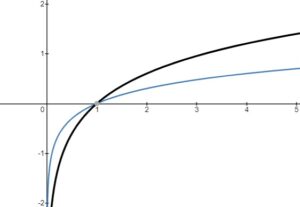 |
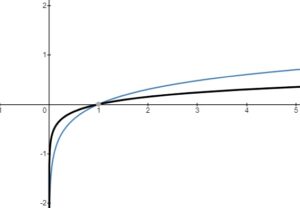 |
 |
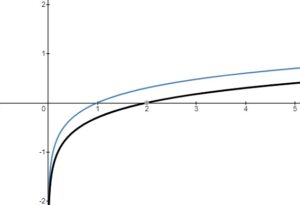 |
 |
 |
 |
 |
 |
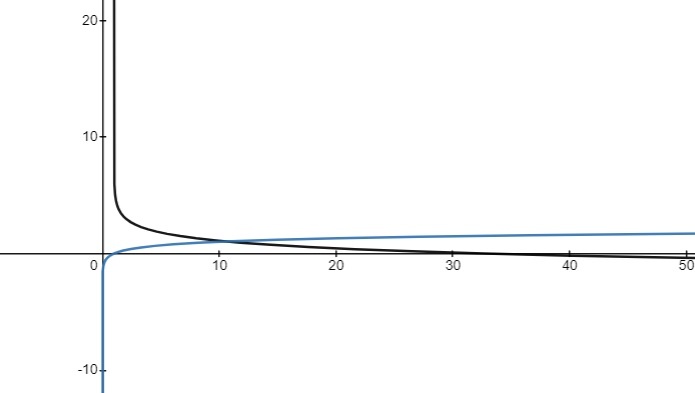
 |
State transformations in order and graph
- vertical compression by a factor of 2
-
reflection in the
– axis
-
horizontal translation 1 unit left
- vertical translation 3 units up
Vertical Asymptote (VA):
– intercept:
– NONE
– intercept(s):
In order to determine the – intercepts, set the equation to = 0, then solve it for
by performing inverse operations in the reverse BEDMAS order.
At the end, the equation would need to be changed to an exponential form in order to be solved.
at this point, in order to solve this equation, represent it in its exponential form, where base is 10 and the exponent is :
Therefore, the – intercept is at (32.6, 0)
Applications of Logs
Log laws (base 10 logarithm)
Example 1: A new car is purchased for $20,000. The car’s value after years is given by
. How long will it take for the car to be worth half of its purchase value?
Solution:
convert into logarithmic form
Therefore, it would take just over 3 years for the car do depreciate to half of its original value.
Example 2: On the Richter scale, the magnitude of the earthquake in City A is 2.7 and the magnitude of the earthquake in City is . How many times stronger is the earthquake in City A?
Formula to compare two earthquakes: where M is the magnitude and I is the intensity.
Solution:
convert into exponential form with base 10
Therefore, the earthquake in City A is approximately 25 times stronger than the earthquake in City B.
Example 3: Tomato juice has a hydronium ion concentration of approximately 0.0001 mol/L. What is its pH? (acidity level)
Formula to determine the pH:
Solution:

Since the pH level of tomato juice is 4, it is acidic.